Introduction to tangent angle circle:
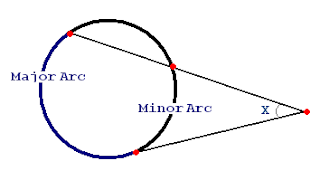
The tangents to a circle are the lines that are from a point outside the circle and intersect the circle at only one point on the circle. When two tangents of a circle are from a single point and angle is formed between the two tangents of the circle. In the following article we will see in detail about the topic tangent angle circle.
More about Tangent Angle Circle:
The tangent of a circle are the lines that intersect the circle at only one point on the circle. If two tangents of a circle arises from a single point outside the circle then there is an angle formed between the tangents of the circle. This angle between the tangents of the circle can be related to the angle covered by the arcs that are formed by the intersection of the tangents with the circle.
Angle between the two tangents from a single point to the circle:
The angle x is the measure between the two tangents is half of the difference between the major arc and the minor arc.
Angle x = (Angle covered by the major arc – Angle covered by the minor arc)/2
Angle between a tangent and a secant from a single point to the circle:
In some cases there can be a tangent and a secant arising from a common point outside the circle in those cases the angle between a tangent and a secant from a point to the circle is half of the difference between the major arc angle and the minor arc angle.
Angle x = (Angle covered by major arc – Angle covered by the minor arc)/2
Algebra is widely used in day to day activities watch out for my forthcoming posts on how do you find the prime factorization of a number and prime numbers 1 to 100. I am sure they will be helpful.
Example Problem on Tangent Angle Circle:
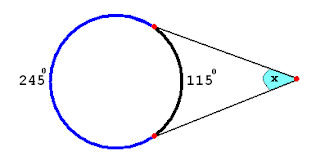
1. Find the angle between the two tangents in the given diagram.
Solution:
The angle x = (Angle covered by major arc – angle covered by minor arc)/2
`= (245-115)/2`
`= 130/2`
`= 65` `degrees`
2. Find the angle between the two tangents to the circle in the given diagram.
Solution:
The angle x = (Angle covered by major arc – angle covered by minor arc)/2
`= (275-85)/2`
`= 190/2`
`= 95 degrees`
Practice problem on tangent angle circle:
1. Find the angle x between the tangent and the secant to the circle in the diagram.
Answer: 50 degrees
The tangents to a circle are the lines that are from a point outside the circle and intersect the circle at only one point on the circle. When two tangents of a circle are from a single point and angle is formed between the two tangents of the circle. In the following article we will see in detail about the topic tangent angle circle.
More about Tangent Angle Circle:
The tangent of a circle are the lines that intersect the circle at only one point on the circle. If two tangents of a circle arises from a single point outside the circle then there is an angle formed between the tangents of the circle. This angle between the tangents of the circle can be related to the angle covered by the arcs that are formed by the intersection of the tangents with the circle.
Angle between the two tangents from a single point to the circle:
Angle x = (Angle covered by the major arc – Angle covered by the minor arc)/2
Angle between a tangent and a secant from a single point to the circle:
Angle x = (Angle covered by major arc – Angle covered by the minor arc)/2
Algebra is widely used in day to day activities watch out for my forthcoming posts on how do you find the prime factorization of a number and prime numbers 1 to 100. I am sure they will be helpful.
Example Problem on Tangent Angle Circle:
1. Find the angle between the two tangents in the given diagram.
The angle x = (Angle covered by major arc – angle covered by minor arc)/2
`= (245-115)/2`
`= 130/2`
`= 65` `degrees`
2. Find the angle between the two tangents to the circle in the given diagram.
The angle x = (Angle covered by major arc – angle covered by minor arc)/2
`= (275-85)/2`
`= 190/2`
`= 95 degrees`
Practice problem on tangent angle circle:
1. Find the angle x between the tangent and the secant to the circle in the diagram.
No comments:
Post a Comment